What does 21st Century math learning look like? Eight Engaging Strategies for the Math Classroom
Often when I met people for the first time and tell them I am a math educator, the majority of the time I receive the reaction “Oh I hated math at school and I’m just not a math person!” This reaction greatly saddens me but is the product of years of being exposed to the drill and kill approach to math learning.
With the exponential growth in technology, knowledge has become a commodity. Our students are able to Google any answer or technique so education needs to focus on teaching students what to do with the knowledge and how to apply and transfer their knowledge to different situations. Math learning should be about developing conceptual understanding in order to prepare our students for the 21st Century. Successful 21st Century math learning needs to foster 21st C skills such as communication, collaboration, critical thinking and problem solving.
Mathematics is a language of conceptual relationships. Traditional pedagogy has focused on rote memorization of facts and skills with little attention paid to the conceptual relationships involved. Facts and skills go hand in hand with conceptual understandings as illustrated by figure 1 below. “In order to develop intellect in our students and increase motivation for learning, curriculum and instruction must create a synergy between the lower (factual) and higher (conceptual) levels of thinking by utilizing the inquiry process continuum” (Wathall, 2014, p14).
Figure 1

Here are my tops tips for engaging your students in the math classroom with the goal of developing conceptual understanding and therefore enhancing learning:
Strategy One: Create a social learning environments that promotes team work and collaboration.
Strategy Two: Provide an open, secure environment to allow for mistakes as part of the learning process. Based on the work of Carol Dweck and Jo Boaler, this strategy includes fostering a growth mindset with your students and colleagues.
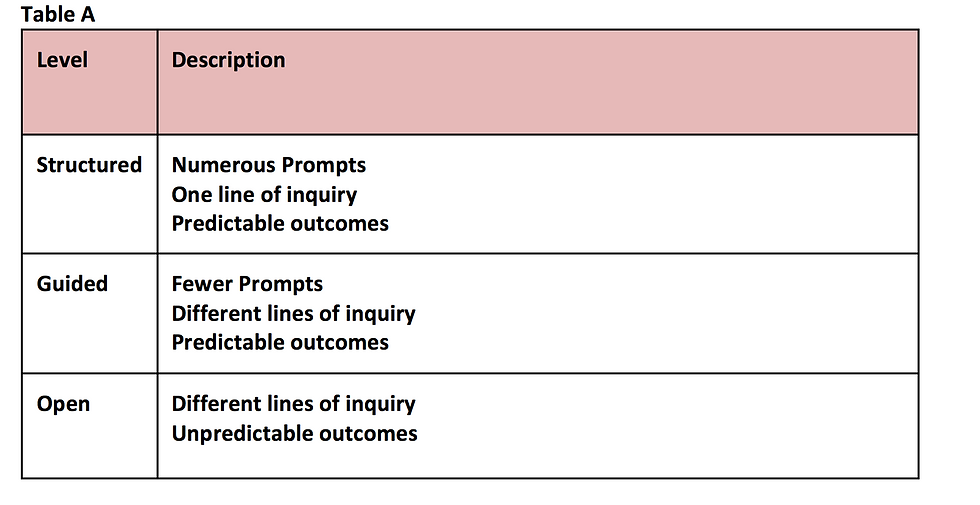
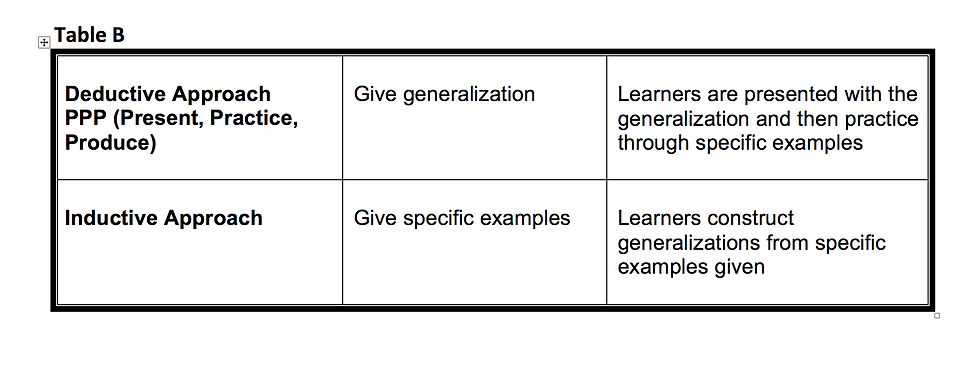
Strategy Three: Use the levels of inquiry (Table A) as described by the table below and employ inductive teaching approaches (Table B). Please see the side by side comparison of a deductive vs inductive teaching approach (Table C).

Strategy Four: Reduce whole class teacher talk time. Brain research shows secondary students can concentrate with direct instruction for around 15-20 minutes.
Strategy Five: Cater to all the needs of your students by differentiating for different backgrounds and interests. Carol Ann Tomlinson’s work on differentiation discusses differentiating by: content, product, process and affect.
Strategy Six: Use Diagnostic, formative and summative assessment. I like to use visible thinking routines from Harvard University’s Project Zero such as “I used to think… Now I think…”
Strategy Seven: Be purposeful when asking students to answer questions. I like to use the thumbs to chest strategy so students can discreetly show whether they have completed a question or would like to answer a question.
Strategy Eight: Flexible fronts and looking at the structure of your classroom. Traditional math classrooms have a “Sage on the Stage” layout which encourages a more didactic approach. Here is a diagram of how I structure my classroom to encourage more collaboration and discussion to promote the “Meddler in the Middle” type of teaching.

Jennifer Chang Wathall is an independent educational consultant specializing in concept-based curriculum and concept-based mathematics. She is author of “Concept-Based Mathematics: Teaching for Deep Understanding in Secondary Schools” and she travels the world facilitating professional development courses and delivering keynote addresses at conferences.





















